The basic task in linear algebra is to solve linear equation systems:

Let's do this symbolically for a  matrix:
matrix:
A = Matrix(3,3,symbols('A1:4(1:4)'))
b = Matrix(3,1,symbols('b1:4'))
x = A.LUsolve(b)
The output of this relatively small problem is already merely readable, which can be seen in the following graphical expression:

Again, the use of simplify command helps us to detect canceling terms and to collect common factors:
simplify(x)
Which will result in the following output, which looks much better:
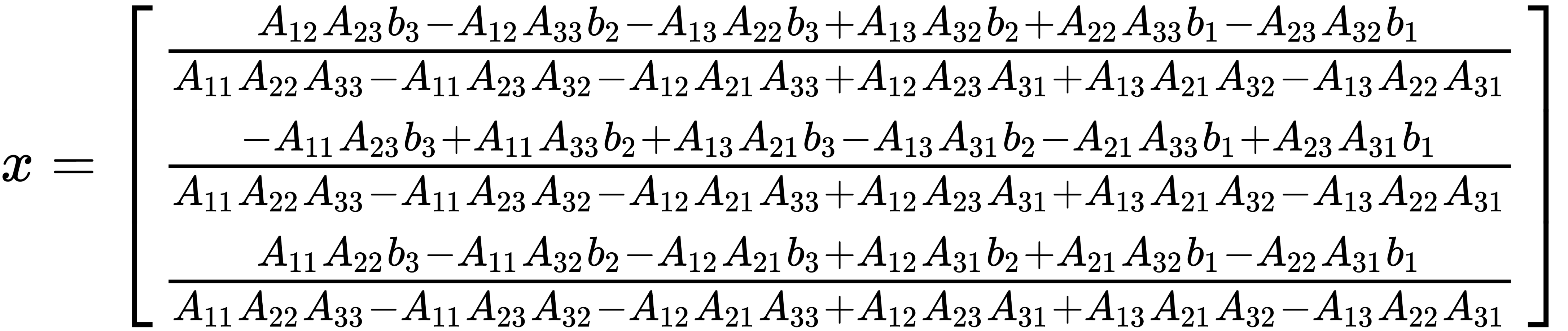
Symbolic computations become very slow when increasing matrix dimensions. For dimensions bigger than 15, memory problems might even occur.
The next figure (Figure 16.3) illustrates the differences in CPU time between symbolically and numerically solving a linear system...
























































